Proof of limit of sin x / x = 1 as x approaches 0

How to prove that limit of sin x / x = 1 as x approaches 0 ?

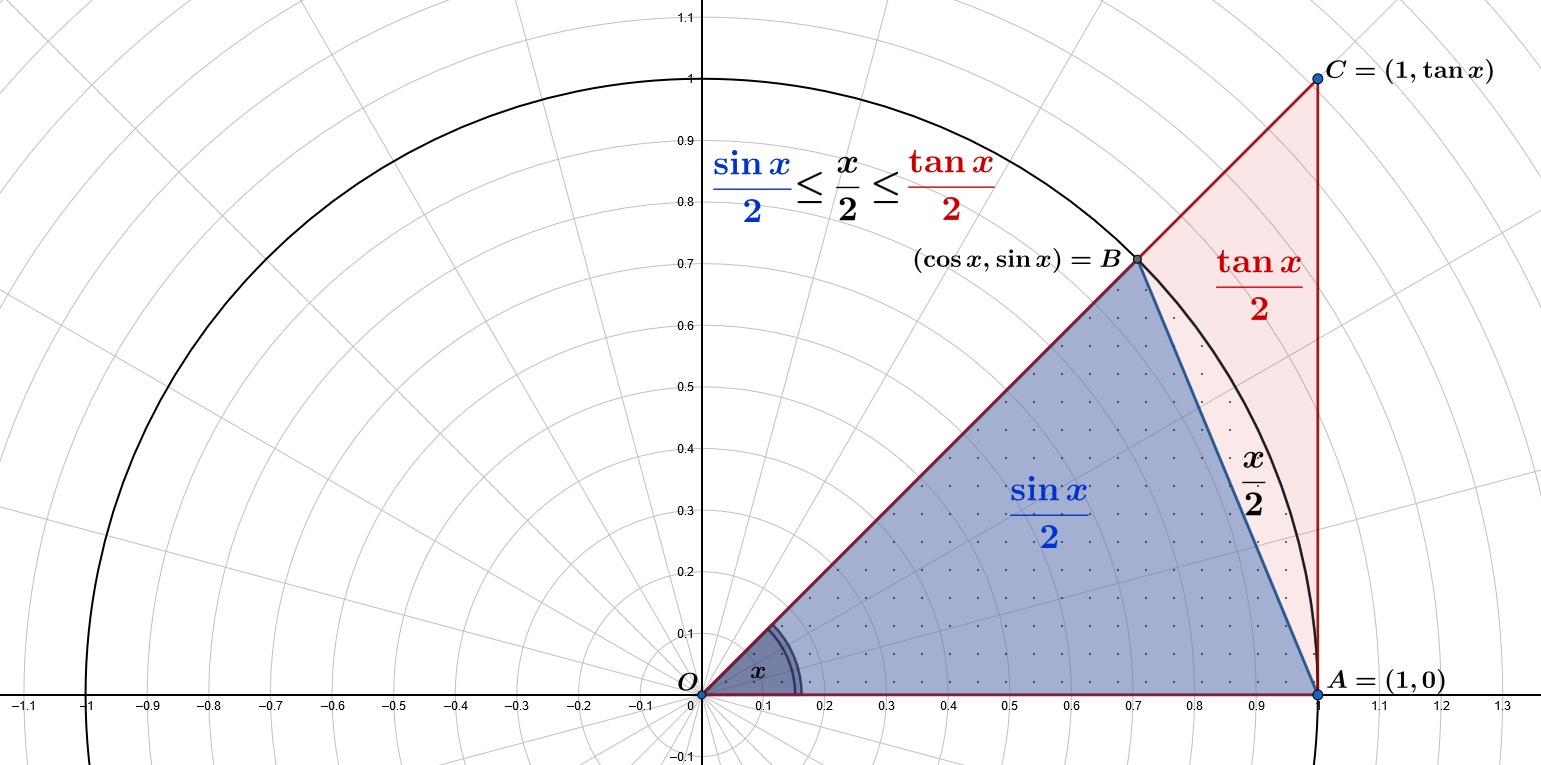
Area of the small blue triangle $OAB$ is ${\displaystyle A(OAB)=\frac{1\cdot \sin x}{2}=\frac{\sin x}{2}}$

Area of the sector with dots is ${\displaystyle \pi \frac{x}{2\pi }=\frac{x}{2}}$

Area of the big red triangle $OAC$ is ${\displaystyle A(OAC)=\frac{1\cdot \tan x}{2}=\frac{\tan x}{2}}$

Then, we have ${\displaystyle A(OAB)\le \frac{x}{2}\le A(OAC)}$:

$$0<\sin x\le x\le \tan x,{\displaystyle \mathrm{\forall }x\in ]0,\frac{\pi }{2}[}$$

Since $0<\sin x$, we have $\begin{array}{rl}\frac{\sin x}{\sin x}& \le \frac{x}{\sin x}\le \frac{\tan x}{\sin x}\\ 1& \le \frac{x}{\sin x}\le \frac{1}{\cos x}\end{array}$

Taking the reciprocal:

$$\cos x\le \frac{\sin x}{x}\le 1$$

Since ${\displaystyle \cos x,\frac{\sin x}{x},1}$ functions are even, then we conclude that:

$$\cos x\le \frac{\sin x}{x}\le 1,{\displaystyle \mathrm{\forall }x\in ]-\frac{\pi }{2},0[\cup ]0,\frac{\pi }{2}[}$$

By using the Squeeze Theorem:

$$\underset{x\to 0}{lim}\frac{\sin x}{x}=\underset{x\to 0}{lim}\cos x=\underset{x\to 0}{lim}1=1$$

we conclude that:

$$\underset{x\to 0}{lim}\frac{\sin x}{x}=1$$